The Probabilities of Sports and Spreads

Cyrus Vanden Bosch

Honors Problem Solving

12-8-18

The Probabilities of Sports and Spreads

          We have all put our luck on the line with probabilities.  A flip of the coin decides who will do the chores.  A roll of the die decides the fate of a game of Monopoly.  In math class we find probabilities that describe the likelihood of an outcome of an event in a word problem.  We like to use definite probabilities because they are fair and we know exactly what our chances are.  We know the chance of a coin landing on heads is one half, and that the likelihood of a die landing on any number is one sixth.

            However, probabilities in sports are practically impossible to calculate precisely.  Human factors create so many variables involved that change the outcome of events.  Bookmakers have computers that run intensive algorithms that process a great amount of information in order to assess what the odds of certain outcomes are.  Obviously in sports some teams are considered better than other.  For games such as American football, bookmakers set spreads for people to bet on.  If the options were to choose who would win the game, people would bet on the better team.  If the bookmakers decide the better team is favored by “x” points, then the spread will be described as such: favored team –x, underdog +x.  Below we see that the Dallas Cowboys are favored by six and a half points over the New York Giants.

          This means that however many points the favored team is figured to win by, the underdog is given on top of their actual score at the end of the game.  If spread is seven points and the underdogs lose by three, then whomever bet on the underdog win as they “covered the spread.”  If they favored team won by ten points, then they have “covered.”  If the favored team wins by exactly seven points then all bets are returned with no profit or loss.  Spreads can be misleading however.  They are not an actual representation of how much better the bookmakers think one team is over the other.  When they set the spreads, their goal is to have an equal amount of people to bet on both sides so they guarantee profits without risk.

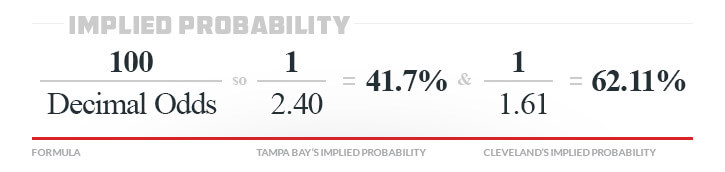
Just getting your predictions right based on this knowledge will not net you a profit safely, as you will never be always correct.  It is best to find the highest value in bets.  This is where implied probabilities come into play.  The decimal odds show you how much you can make through a wager by betting any amount.  If the odds of a team winning are 2.50 and you bet 10 dollars, multiply these together and you will get the return of $25 for a win.  Implied probability is what the odds suggests as the likelihood of outcomes are.  You calculate it by dividing one by the decimal odds.  If the odds of a team winning is 2.50, then the implied probability is 1/2.50 or .4 (40%).  An example of the implied probabilities for a football game:

From here we can calculate expected value.  If the Detroit Lions have odds of 2.5, and you wager ten dollars on them, you can expect a 25 dollar return if you win.  If they lose, you lose the 10 dollars.  You can expect to make $15 in profit 40% of the time and lose $10 60% of the time.  Expected Value = (Probability of Winning x Amount Won per Bet) - (Probability of Losing x Amount Lost per Bet).  For the Lions scenario: (40% x $15) - (60% x $10) = $6 - $6 = $0.  The expected value of this bet is zero, so in the long run making this wager should you keep you about even. 

Here is where the bookmakers get you.  If you add the two implied probabilities together you will get a number greater than 1 or 100%.  This value represents the advantage of the sportsbooks.  Making the odds greater for each team means they will draw more money, owe less to winners, and make more profit.  The implied probabilities the bookmakers give are greater than the real probability, so the expected value is negative and should be considered a poor bet.  Bettors try to bet on games with percentage values as close to 100% as possible in order to lower the advantage of the sportsbooks.  The goal should be to bet on games where you think the real probability is greater than the implied one, so the expected value is positive and you will win in the long run.  This is where sports knowledge and recognizing data and trends comes into play, because good wagers involve betting on an outcome where you think you have the advantage over the booksmakers with a positive expected value.

For example, in college football Iowa generally plays very well at home against really good teams in the Big Ten that on paper should dominate them: let’s take Michigan and Ohio State.  The last time Iowa played Ohio State, Iowa blew the Buckeyes out 55-24 at home.  The Buckeyes were 17-point favorites in that game.  The last time Iowa played Michigan, the Hawkeyes beat the Wolverines 14-13 in a close home game.  The Wolverines came into that game as 18.5-point favorites.  From these examples, it is clear that Iowa will show up during big home games and will keep it close, if not win, doing so in stunning fashion against Ohio State in 2017.  If I were to see a solid good team going on the road to Iowa, especially at night, I am choosing Iowa to beat the spread if they are big underdogs. 

Another great example is Michigan vs. Northwestern in basketball.  In past year these games have been very close and go either way all the time.  On December 4th, 2018, FanDuel had the Wolverines on the road as five point favorites.  This season the Wolverines have blown out all of their opponents in stunning fashion, with their lowest margin of victory being 17 points.  They blew out the two teams (North Carolina and Villanova) that have comprised the last three years’ national champions.  You would think the Wolverines would easily cover the five point spread against the relatively lesser Wildcats, and most people did, 80% of them betting on the Wolverines.  However Michigan ending up winning the game by only two points in a 62-60 nail biter.

Two lessons should be learned here.  First, knowledge of sports and trends are vital when making predictions.  Second, don’t gamble, the sportsbooks always win and it is not easy to make money without being at great risk.  Sports have so many variables involved, and only the most knowledgeable and experienced people should think about wagering their money.

Works Cited

Moody, Allen. “How Do Points Spreads Work?” Thoughtco., Dotdash, www.thoughtco.com/sports-betting-understanding-point-spreads-3116853.

Moody, Allen. “What Is the Point Spread in Sports Gambling?” Thoughtco., Dotdash, www.thoughtco.com/how-the-point-spread-is-made-3116858.

“Basic Probability & the Concept of Value.” GamblingSites.org, www.gamblingsites.org/sports-betting/beginners-guide/basic-probability-and-value/.